Keywords: learning, differential equations, physics, models.

Abstract

This article presents a bibliographic review of the topic “Differential equations applied in physics”, from a didactic approach, to expose the main authors and contributions that have been given to the sciences. Regarding the methodological part, a content analysis was made, through a documentary-bibliographic review of studies published between 2011 - and 2021. Relevance, language, year of publication, and place of origin were used as thematic criteria when selecting the suitable material to be analyzed. As for the sources of reliable information, the academic Google search engine was used. After performing the search, a bibliometric and qualitative content analysis was carried out to select the most relevant publications for this study.

At present, higher-level mathematics is still considered difficult and not very applicable in real life, especially when dealing with differential equations, where calculus topics such as derivatives are taken, which, when taken to physics, are considered very complex to analyze due to the fact of having to deal with a physical and mathematical model. There is a wide variety of ways to train students’ learning through active methodologies and more in-depth studies, which approach the modeling of equations from an abstract point of view.

The results indicate that the learning of differential equations in physics is very little and by increasing the part of the use of technology to acquire professional competencies, the number of publications in specialized articles becomes smaller.

I. Introduction

For teachers of mathematics and physics, finding alternatives means to continue improving their educational work, which has been a constant that has transcended over time. Finding didactic models that allow the motivation of students, to achieve the acquisition of new skills, taking advantage of every one of the resources available, so that the class has a better acceptance and understanding, despite its level of complexity.

Education in general, and higher education in particular, has faced different changes and challenges throughout its history, as a product of social needs and demands, which incite in some way to adapt to the new realities of the 21st century. (Roa, 2021, p. 65).

Few studies are referring to technology, differential equations, and physics, making it very difficult to put together this tern, therefore a search was made in different works related to it, even at the national level no antecedent was found, being in the area of applied mathematics more worked the general topics as in the doctoral thesis of Blandón (2017), entitled “Methodological proposal for the teaching-learning process of the Algebra unit in the subject of General Mathematics in the Multidisciplinary Regional Faculty FAREM-Estelí” in which aspects related to epistemology and conceptualization are collected to create a proposal of general aspects.

Being the subject closest to differential equations the doctoral thesis presented by Granera (2017), entitled “Teaching-learning process of the integral defined as the area under a curve in the subjects of Calculus in Engineering majors. Study conducted at the Multidisciplinary Regional Faculty of Estelí” which proposes a review of the integral term as a starting point in its theoretical references, for the development of a proposal based on computational environments.

Both mathematics and physics are among the great sciences in which man has tested and tried to explain the reality of the various phenomena that occur in everyday life, approaching models such as mathematics or physics. Grounded theory is used in this study since it is an approach based on theoretical development in the information collected and analyzed systematically. Its main characteristic is the constant comparison method; it is a process of inductive analysis in which information and data analysis interact until they are reduced to the most representative concepts.

The physical model can refer to the theory building (mathematical model) of a physical system. Likewise, there are simulations of everyday phenomena, which have aspects of the behavior of complex physical systems, where different laws, equations, and the so-called physical analysis are taken into account for the understanding of science.

II. Methodology

The implementation of this review article is based on a qualitative paradigm, from an interpretative approach, given its orientation to content analysis, under a bibliographic documentary methodology. As an information search strategy, sources continued to be identified, selected, and categorized by topic, year of publication, and relevance between 2011 and 2021. These are related to the topic of differential equations applied in physics. In addition, studies obtained from other Internet sources through Academic Google were considered valuable to contribute to the research and were also included in this study.

A narrative review was used to analyze the data obtained, since this is a strategy that facilitates the understanding of the subject matter on the application of differential equations in physics, with learning by competencies, since it describes it with a theoretical foundation and includes different types of information, considering different sources and enabling learning through the definition and detail of concepts.

The categories of analysis were: differential equations, competencies, and didactic methodologies for learning mathematics.

III. Results

Table 1

Research according to the categories of analysis

Note: Own production

Regarding the distribution according to the categories of analysis, it is evident the frequency of articles produced mostly in the area of didactics of mathematics, mostly using technology, since learning difficulties are frequent and with technological resources, the student finds the class attractive, for competencies only 25% of articles were found, this is mostly because recently many universities went from a system by objectives to one by competencies, and in the area of mathematics and physics, very few authors have dabbled in writing.

12.5% of the articles refer to differential equations, in their most abstract and demonstrative form, where their application is seen in the different branches of the natural sciences and specifically in Physics, this is since it is a somewhat complex subject, due to the analysis of the different scientific models.

Table 2

Distribution of items by country of origin

Note: Own production

The greatest scientific production in terms of the subject under study is in South America and Mexico because in those locations many methodologies are implemented to achieve significant learning through the acquisition of mathematical competencies. In second place Nicaragua and Cuba, because sometimes the studies carried out are not published and remain only in physical form in libraries.

Lastly, Spain, although they publish annually in different journals of impact, has not had many recent publications on differential equations.

IV. Discussion of the Results

In recent years, there have been many scientific publications, referring to methodologies for learning differential equations, applied in physics, where it is added the use of technology, which is an important competence in today’s world.

To understand more about the subject, we start with a preamble about derivatives, as this is one of the main concepts of differential equations.

For (Zill, 2018) the derivative dy ⁄ dx of a function y = ϕ(x) is another function ϕ'(x) found with an appropriate rule. The function 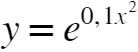is derivable on the interval (-∞,∞) and using the chain rule, its derivative is 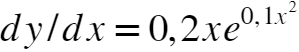(p.2)

If 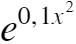is substituted into the right-hand side of the last equation for y, the derivative will be

Equation (1) is called a differential equation, but it is necessary to give a formal definition:

An equation that contains derivatives of one or more variables to one or more independent variables is said to be a differential equation (DE). (Zill, 2018, p. 2)

Once differential equations have been defined, mention can be made of the works that have been carried out at the international level, referring to the subject under study.

Guzmán (2021) conducted a doctoral thesis entitled: Didactic model for the development of competencies in differential equations in engineering students in a public university of Lambayeque in Chiclayo, Peru.

The development of competencies is an important factor in the formation of university students since it implies training them in capacities, skills, and aptitudes that allow them to build their knowledge and achieve significant learning (Guzmán, 2021, p. 11).

The research started with the diagnosis of a sample of 50 students of the fifth cycle, to whom a questionnaire was applied to measure the development of competencies in the didactic unit of differential equations, they obtained a poor level of achievement, which showed that they fail to develop the competencies indicated in the didactic unit at issue, in this situation this study proposed a didactic model, which was based on learning theories and socio-training approaches, which was validated by the judgment of experts who determined its relevance and applicability.

The learning of differential equations is always complex because it has a certain numerical and algebraic complexity, which is reflected when the number of students is large and they do not harmoniously achieve competencies; on the contrary, it becomes frustrating, when the teacher is seen as the villain of the educational process.

The aforementioned complexity is sometimes manifested when combining differential equations with applications to physical problems, for example for Abalos (2019):

Nonlinear electrodynamic theories arise from arbitrary Lagrangian in terms of electromagnetic invariants. These theories present dispersion relations defined in terms of two effective Lorentzian metrics. Where it has been proved that these theories are hyperbolic symmetric (a class within the strongly hyperbolic ones) if and only if, the time cones of those effective metrics have nonempty intersection. (p.60).

4.1 Application of equations in physics

For Herrera (2021) “physics allows the development of analysis, interpretation and synthesis skills through scientific, methodological and investigative knowledge related to Physical Science that makes it capable of performing in the work environment”. (p.15).

For Mañas and Martínez (2015):

Physics is full of linear and nonlinear Partial Differential Equations (PDEs). In both electromagnetism and quantum mechanics, the basic equations are linear, but in other areas, such as the dynamics of continuous media or general relativity, the fundamental equations are nonlinear. (p.5).

There are four fundamental examples of linear PDEs, all of which are of second-order and are mentioned below:

4.1.1 The Poisson equation

Where f = f ( x, y, z ) is a given function. If f ≡ 0 the PDE is known as Laplace’s equation. Both PDEs appear often in electrostatics and fluid mechanics. (Mañas and Martínez, 2015, p. 5).

4.1.2 The wave equation

Where is c a positive real number representing the propagation velocity of the waves. (Mañas and Martínez, 2015, p. 5).

Where is c a positive real number representing the propagation velocity of the waves. (Mañas and Martínez, 2015, p. 5).

4.1.3 The Schrödinger equation.

Which describes the dynamics of a particle of mass m in a force field with potential function q = q ( x, y, z ). The symbol ℏ represents the normalized Planck’s constant. It is to be noted the presence of the imaginary number i in the coefficient of . (Mañas and Martínez, 2015, p. 5).

4.1.4 The heat equation

It is relevant in thermal diffusion and fluid diffusion processes in general. The symbol 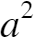represents the diffusion coefficient. (Mañas and Martínez, 2015, p. 5).

To write in abbreviated form the above equations it is convenient to use the notation of the Laplacian operator

Thus it is obtained:

1. Poisson equation in 3 dimensions:

2. Wave equation in 1+3 dimensions:

3. Schrödinger equation in 1+3 dimensions:

4. The heat equation in 1+3 dimensions:

Simplified versions of the above equations are sometimes considered in which u does not depend on some of the variables ( x, y, z ) Thus, a 1 + 2 dimensional version of the wave, Schrödinger or heat equations is a PDE in which u is assumed to depend on ( t, x, y ) only. (Mañas and Martínez, 2015, p. 6).

For Mañas and Martínez (2015) there are many examples of nonlinear PDEs of great importance for their applications in Physics. The methods used in their study are very different from those developed for linear PDEs. (p.6)

Some examples:

1. The Korteweg-de Vries equation.

With applications in hydrodynamics, solid-state physics, and plasma physics. (Mañas and Martínez, 2015, p. 6).

2.The nonlinear Schrödinger equation

Relevant in various fields among which nonlinear optics stand out. (Mañas and Martínez, 2015, p. 6).

It is important to mention that Bel (2014) carried out a thesis entitled: Oscillatory solutions in Differential Equations with delay.

The author uses Differential Equations with delay to model problems in Physics, engineering or biology, among others. These equations are an example of functional differential equations. The complexity present in these models is much greater than that observed in ordinary Differential Equations, it is enough to consider a first-order equation in one dimension that includes a parameter and a delay, to find periodic motion, quasiperiodic or chaotic behavior.

The first methodology presented in this thesis combines the use of the homotopic analysis method and a collocation method to calculate the stability of existing periodic cycles. The advantages presented by this procedure and the various adaptations made make it possible to describe interesting dynamic scenarios in different equations with delay. The author analyzed the Van Der Pol equation where fed back with delay, different bifurcations, and resonances involving one or several periodic cycles.

Undoubtedly, the subject of differential equations is wide, but when dealing with its applications in physics it becomes something complex to understand by university students, this because the “repetitive” and not “analytical” part is deeply rooted, that is to say, students only repeat given steps, but it becomes difficult when they must analyze a phenomenon that occurs and get to use physical and mathematical models.

Although there is an intention to improve the teaching work in university classrooms, some teachers still have the idea of teaching Mathematics only with a Calculus book as the only means, placing directly the definitions and solving exercises from it, without coherent planning that offers the student the opportunity to understand the definition and to know how it was reached and what it is for. Further deepening this problem, the almost null involvement in teaching, technological means, and environments relevant to the interests of students cause failure in the subject. (Flores, 2017, p. 36).

4.2 Use of technological resources

Virtual classrooms are a vitally important factor in the transformation of various fields of society. Technological tools have the potential to transform the nature of education in terms of where and how the teaching-learning process occurs, as well as to introduce changes in the roles of teachers and students, and the different actions performed in the educational process. (González and Granera, 2021, p. 51).

The learning of Mathematics and Physics should be seen as a specific and complex activity in which scientific knowledge is not enough, but also teachers who facilitate it use didactic resources that motivate students. A poorly designed Differential Equations class is detrimental to students in the long run since from their point of view it becomes boring and turns into a punishment where they are graded, judged, and even scolded, especially if these students will be the future transmitters of knowledge in the classroom.

The accelerated advances of information and communication technologies (ICT) have marked the before and after, allowing the participation of all sectors of society. The incorporation of these technologies in education has revolutionized how we educate ourselves and changed personal and academic life, which has made available a wide range of integrative and participatory tools for the construction of a new society. (Fletes, 2021, p. 2).

Studies at the doctoral level that have linked mathematics and virtual environments are many, but they fail to bring together the tern, differential equations, physics, and technology.

For Potosme (2017).

To talk about ICT is to allude to electronic media, satellite and cable television, cellular telephony, computers, and software that facilitate access to networks, essentially because technological advances have given the computer a leading role as a pedagogical resource, generating a resounding change, by allowing the development of activities where access to different sources of information can be had. Particularly in Mathematics, the possibilities of teaching and learning are superior since it is possible to access different application programs that allow visualizing the geometric representation, for example, of abstract objects, reducing time in the execution of large calculation problems, as well as motivating the students. (p.67)

The use of technological media requires a new configuration of the teaching process and methodology because the content to be developed does not have to be completely in the hands of teachers, and students are no longer just receivers of information, they are now builders of their learning.

For Padilla, et al (2014) the appropriation of ICT in higher education brings with it a whole interpretative perspective on the importance of pedagogical discourse to address the difficulties of interaction, roles, and flexible pedagogical possibilities in the framework of the information society. (p. 272)

It must be understood that ICTs are not just a simple tool, but in the first place constitute a new form of dialogue, aesthetics, narrative, relational connection, identity, and worldview. One of the consequences is that when a person is excluded from access to and use of ICTs, the form of existence and existence of the world will be lost, and others will also lose these contributions.

Regarding the use of technologies, by incorporating them into the subject of Mathematics, they offer a wide variety of resources and applications to teachers. They can be used as tools for performing complex calculations, solving problems, drawing graphs, and interpreting and analyzing data. This means, a convenient aid for the improvement of the teaching-learning process and particularly of Mathematics, of course, this is achieved through an appropriate application of the same, which has allowed the author of this study, to perform an analysis of virtual environments as a resource for learning Mathematics. (González and Granera, 2021, pp. 58-59).

Technology has been used as a means of verification for highly complex calculations, but the student must have sufficient analytical skills to interpret the results obtained.

Consequently, from all the above described, in the results presented by the authors of the different researches selected and analyzed, the importance of the subject of differential equations applied in physics in the process of teaching and learning Mathematics is evidenced, considering the use of technology as a support to improve the quality of education.

V. Conclusions

This review article has made it possible to clarify several points. Firstly, there are few studies of differential equations that relate to physics and technology, taking into account that the latter cannot be seen as a substitute for teaching.

The applications of differential equations in physics are many, but sometimes they are not seen in classroom processes because they are focused on the repetition of mathematical steps.

The use of technological resources should be seen as an additional strategy to achieve, on the one hand, to motivate students to experiment with applications through software, simulations, and interactive tools.

It is necessary to create methodologies related to the application of differential equations in physics, using technology, to contribute to the teaching-learning processes at the university level.

The use of technological resources leads to providing a solution to the demands of today’s society allowing to promote of the creativity of both teachers and students through the use of all the potential offered by these technological tools.

Glossary of technical or specialized terms

---

VI. Bibliography

---

Blandón Dávila, M. E. (2017). Propuesta metodológica para el proceso de enseñanza-aprendizaje de la unidad de Álgebra en la asignatura de Matemática General en la Facultad Regional Multidisciplinaria FAREM-Estelí, UNAN-Managua. Tesis para optar al grado de doctora en Matemática Aplicada, Estelí, Nicaragua. Recuperado el 5 de Marzo de 2021, de https://repositorio.unan.edu.ni/8818/

Fletes Calderón, O. R. (2021). Las nuevas tecnologías en la educación superior, una oportunidad. Revista Torreón Universitario, 1-2. Recuperado el 3 de Enero de 2022, de https://revistatorreonuniversitario.unan.edu.ni/index.php/torreon/article/view/362/661

Flores Morales, J. J. (2017). Modelo para la Enseñanza y Aprendizaje Asistido de las Matemáticas:Una propuesta didáctica de microplanificación para el desarrollo del tópico interpretación geométrica de la derivada y sus implicaciones en el análisis funcional. Universidad Central “Martha Abreu” De Las Villas Santa Clara, Cuba Y Universidad Nacional Autónoma De Nicaragua Unan Managua, Juigalpa, Nicaragua.

González, J. I., y Granera, J. A. (2021). Entornos Virtuales de Aprendizaje (EVA) para la enseñanza-aprendizaje de la Matemática. Revista Científica de la FAREM-Estelí: medio ambiente, tecnología y desarrollo humano, 49-62. Recuperado el 2 de Diciembre de 2021, de https://rcientificaesteli.unan.edu.ni/index.php/RCientifica/article/view/1080/1142

Granera Rugama, J. A. (2017). Proceso de enseñanza–aprendizaje de la integral definida como el área bajo una curva en las asignaturas de Cálculo en las carreras de Ingeniería. Tesis presentada para optar al título de Doctor en Matemática Aplicada, Facultad Regional Multidisciplinaria, Chontales, Estelí, Nicaragua. Recuperado el 14 de Marzo de 2021, de https://repositorio.unan.edu.ni/8823/

Guzmán Roldan, C. M. (2021). Modelo didáctico para el desarrollo de competencias en ecuaciones diferenciales en estudiantes de Ingeniería en una universidad pública de Lambayeque. Tesis Para Obtener El Grado Académico De: Doctora en Educación, Universidad César Vallejos, Chiclayo, Perú. Recuperado el 13 de Diciembre de 2021, de https://repositorio.ucv.edu.pe/handle/20.500.12692/63824

Herrera Castrillo, C. J. (2021). prendizaje en las asignaturas “Electricidad” y “Termodinámica y Física Estadística” en tiempos de pandemia. Revista Multi-Ensayos, 14-25.

Mañas Baena, M., y Martínez Alonso, L. (2015). Ecuaciones Diferenciales II. Manual, Universidad Complutense de Madrid, Departamento de Física Teórica II (Métodos Matemáticos de la Física). Recuperado el 20 de Diciembre de 2021, de https://eprints.ucm.es/id/eprint/31464/

Potosme Mercado, N. G. (2017). Análisis de la incidencia de la Unidad Didáctica sobre la integral definida, en la conceptualización que tienen los estudiantes de segundo año de la Carrera de Contaduría Pública y Finanzas de la Universidad Politécnica de Nicaragua (UPOLI). Tesis presentada para optar al título de Doctor en Matemática Aplicada, Facultad Regional Multidisciplinaria De Chontales, Juigalpa, Chontales. Recuperado el 1 de Mayo de 2021, de https://repositorio.unan.edu.ni/8826/

Roa Rocha, J. C. (2021). Importancia del aprendizaje significativo en la construcción de conocimientos. Revista Científica De FAREM-Estelí, 63–75. Obtenido de https://www.camjol.info/index.php/FAREM/article/view/11608/13465

Zill, D. G. (2018). Ecuaciones diferenciales con aplicaciones de modelado (11 ed.). (A. E. García Hernández, Trad.) Cengage Learning Editores, S.A. de C.V. Recuperado el 22 de Diciembre de 2021, de https://issuu.com/cengagelatam/docs/zill_am_issuu