Keywords: Prototype; continuity; vectors; integrals; differential

Abstract

This paper aims to present a prototype of experimental work that demonstrates the continuity equation in its differential and integral form, considering vectors. Which allows the development of capacities, skills, abilities, attitudes, and values necessary to obtain, interpret, and process information. Experimental work prototypes should be designed in a way that addresses content effectively. This study is aimed at researchers, teachers, and students from various engineering, physics, and mathematics careers, as it will be of great help in understanding the fundamental principles of mass conservation in fluid systems, something common in the field of fluid dynamics and fluid mechanics. The line of research focuses on the application of exact sciences, physics, and applied mathematics, to establish links between different subjects. This study uses a mixed approach, combining qualitative and quantitative elements to gain a complete understanding of the topic. Techniques typical of this type of research are used, such as the review of documents, books, and journals, addressing each of them individually to establish generalities. The research group is committed to evaluating this work using an evaluation rubric, which ensures its reliability and effectiveness for other researchers and people interested in the topic. It is expected that the results obtained will contribute to knowledge in this scientific field.

1. INTRODUCTION

The present research work tries to demonstrate through a prototype of experimental work the continuity equation in its differential and integral form, when considering vectors, and thus be able to analyze theoretical concepts of this equation. In addition, we worked with easily accessible materials such as cardboard, plastic containers, and wood for the construction of the prototype. Of the above, it was decided to delve into both physics and mathematics topics, since the different subjects are linked, thus being an interdisciplinary tool.

It is necessary to mention that the prototype being a high-dimensional tool, it is applicable in different disciplines, in this case, particularly in Physics and Mathematics, but it can be perfectly modified according to the circumstance that merits it. In addition, it will be of great help to teachers since it will facilitate the explanation of certain contents and thus satisfactory learning will be obtained.

To Vázquez Méndez et al. The use of an experimental prototype is essential to demonstrate and validate the continuity equation in its differential and integral form. This tangible model allows experiments to be conducted and empirical data to be collected to support the conservation of mass in fluid systems. Through its construction and application, abstract concepts are converted into tangible realities, facilitating the understanding and practical application of the continuity equation in fluid dynamics and fluid mechanics. (2024)

The practical experimental work in this research is a fundamental tool in the teaching-learning process in the Experimental Sciences, specifically in Physics and Mathematics, which constitutes an opportunity for students to innovate and develop research capacities and skills, for the demonstration of the continuity equation.

Experimentation and observation have been vital to the development of modern science. Ortis Narváez (2016)states “It is the teaching-learning process of science, the practical-experimental activity is a pedagogical activity that has as its main purpose the apprehension by the students, for which the means of the activity are used” (p. 18). These make the difference between the ancient and the modern era, which is why today’s science cannot be separated from experimentation (practical-experimental work).

Table 1.

Types of Prototypes of Experimental Practical Work

Taken from (Jimenez Cano, 2018)

In addition, it is important to note that this research aims to disseminate knowledge in the field and allow other people, especially students of various engineering, physics, and mathematics careers, as well as teachers who are looking for reliable information for their classes, to benefit from thisz work. Given that there is little research available on this specific topic, this work acquires significant relevance for the entire educational community. Its application can encourage a multidisciplinary approach by addressing concepts related to the continuity equation, thus promoting a more complete and practical understanding of the fundamental principles of conservation of mass in fluid systems.

This study has vital importance to understand the behavior of fluids in pipes and to describe the associated vector magnitudes. Through the implementation of an experimental prototype, the flow of water is simulated, considering its incompressible and unidirectional character. This research will have a positive impact by providing accurate and relevant information that will strengthen the development of the project in question. It will also make it possible to efficiently and effectively address the challenges and aspects related to the topic under study.

In the field of fluid mechanics and practical experimental work, several studies have been carried out that address different aspects. For example, Delgadillo Tijerino et al. They developed a prototype of experimental practical work to demonstrate Euler’s equation and the principle of conservation of energy using integrals and vectors, using a hydroelectric plant as a case study. On the other hand, Mairena Mairena et al. They carried out an experiment to demonstrate the existence of miscible fluids from the vector calculus approach, analyzing sequentially and logically the theoretical concepts related to integrals, vectors, fluid mechanics, and prototypes. Talavera et al., on the other hand, undertook a prototype of experimental practical work to demonstrate Pascal’s principle. (2023)

In addition, research has been carried out focused on the writing of unpublished problems. López López et al. They explored the applications of integral calculus in fluid compressibility in a vector field, using concepts and theorems to solve novel problems. Ponce Herrera et al. They worked on the implementation of the defined integral to analyze the viscosity of fluids, adapting problems extracted from a work by Víctor Streeter. Ortuño Blandón et al. They proposed situations related to the analysis of the definite integral to calculate the magnitudes, forces, and pressures of fluids at rest. (2023)

Likewise, research has been carried out that focuses on the use of simulators and mathematical assistants to address concepts in fluid mechanics. For example, Muñoz Vallecillo et al. They demonstrated Pascal’s principle using integrals and vectors, employing simulators such as PhET and a hydraulic press. These technological resources facilitated the understanding and analysis of this fundamental principle of fluids through natural and common methods. In a similar vein, Cornejo Casco et al. used the PhET simulator to demonstrate the continuity equation from a differential and integral perspective, including vector treatment. This research demonstrates the value of mathematical simulators and assistants as effective tools for teaching and understanding key concepts in fluid mechanics. (2023)

These studies contribute significantly to the advancement of knowledge in the field of fluid mechanics, providing practical approaches, concrete applications, and technological resources that enhance the understanding and analysis of fundamental principles related to fluids.

2. METHODS

2.1. Type of Study

This research is descriptive since its main objective is to collect data and information to demonstrate through a prototype of experimental work the continuity equation. The analysis of the scope of this work allows us to conclude that it is essentially a descriptive study, since “it can be developed with a quantitative or qualitative approach” (Valle Taiman et al., 2022, p. 15)

According to Abreu:(2012)

Descriptive research fits into the two definitions of research methodologies, quantitative and qualitative, even within the same study. Descriptive research refers to the type of research, design, and data analysis question that applies to a given topic. Descriptive statistics answer the questions of who, what, when, where, and how. (p. 192)

2.2. Approach

The focus of this research is mixed, as stated by Sánchez et al. A mixed methodology is used that integrates both quantitative and qualitative data. Regarding the qualitative approach, data were collected and the information obtained was analyzed. In addition, it is important to note that this research also incorporates elements of the quantitative approach, as data collection and analysis are used to answer the research questions posed. (2022)

2.3. Paradigm

This research has an interpretative paradigm, since it concentrates on its object of study, in addition to very important qualitative techniques. It should be noted that the aim of the research is the construction of practical theories.

According to Ricoy Lorenzo (2006)”Interpretative paradigm is considered as interpretive symbolic, qualitative, naturalistic, humanistic and phenomenological” (p. 14).

2.4. Collection of Information

This research is documentary, it is necessary to search for sources of information, in this case of the secondary type. Specialized journals, books, and websites that provide information about the continuity equation in its differential and integral form, taking into account vectors, are used. Documentary analysis is a process created to organize and represent the knowledge that is recorded in documents.(Herrera Castrillo, 2023)

According to Osorio and Añez: (2016)

Secondary sources of information: refer to information resulting from the review, analysis, and synthesis of primary documents. Secondary sources are books and articles that interpret other works or research. Reviews of primary documents based on meta-analyses that involve the use of inferential statistics, which are considered primary sources, are accepted. (p. 110)

2.5. Analysis of Information

The analysis of this research was based on the review of documents as a data collection technique, which was relevant to the development of the study. In addition, a qualitative approach was adopted and various instruments were used to carry out a methodological proposal that includes the construction of a prototype to demonstrate the continuity equation in its differential and integral form, also considering the vectors. The evaluation of the process will be carried out through the design of a rubric that will assess both individual and teamwork. In summary, the analysis of the information is carried out through the implementation of a practical experimental approach.

3. ANALYSIS AND DISCUSSION OF RESULTS

During the process of analysis, evident, positive results were obtained on theoretical concepts of the continuity equation in its differential and integral form, when vectors were taken into account through a prototype of experimental work. In addition, a table was used to triangulate the information on possible solutions to provide a solution and at the same time understand the conceptual aspect of this topic.

Table 2.

Content Analysis, Possible Solutions

Own Creation

When analyzing the theoretical concepts of the continuity equation in its differential and integral form, when considering vectors, it can be seen that these are better appreciated, using experimental practical work that leads to a better perception of the real. This is of great importance since what is conceptualized is practically reflected, that is, from the scientific to the practical and vice versa. Based on the prototype, specifically in the experiment, the concept of continuity equation was analyzed, which states that, if there is no loss of fluid within a uniform pipe, the amount of flow that enters will be equal to the amount of flow that leaves in each time, regardless of its diameter.

In Figure 3D, the mathematical proof of the continuity equation was analyzed, since it makes a graphic reference emphasizing the largest and smallest area of a uniform tube where the parts that form it are identified. In addition, it was possible to interpret the fluidity of a flow through the 3D cube, but taking the three dimensions x, y, and z as a reference, thus demonstrating the differential and integral form of the continuity equation, taking up Reynolds’ Theorem, since it states that in a closed body no mass enters or leaves so there is no loss, therefore, the 3D cube gives graphic emphasis to the aforementioned ones.

During the construction of the prototype of experimental work, information from several reliable sources and accessible materials was required, in addition to analyzing respectively the theme to be demonstrated. It is also necessary to develop the appropriate prototype for the content, which is a fundamental tool for the expression of the continuity equation.

Figure 1.

Prototype Construction Materials

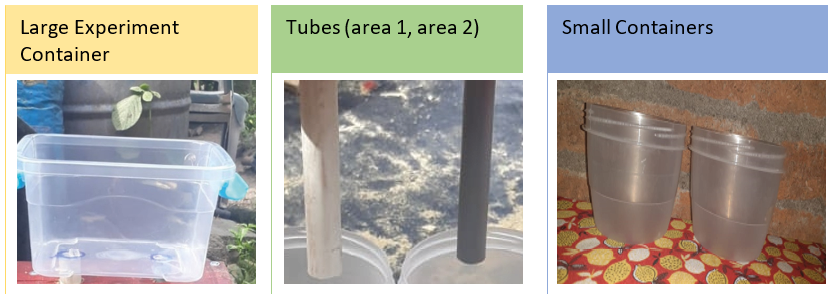
Own Creation, obtained from SmartArt 2024

The experiment consists of a large container, which will serve as a conductor and represents the inlet area of the water that will be distributed on its base where there are two tubes of different diameters which refer to both area 1 and area 2, these being the outlet area, the water will take that direction in a certain time, It falls on two small containers, one for each tube, thus visualizing that both containers receive the same amount of water, regardless of the difference in diameters of the tubes. It is worth mentioning that during the experimentation it was also appreciated that the velocity of the smaller diameter tube is greater than that of the larger diameter, this is due to the pressure since the narrower tube exerts greater force and in the vector part, it is taken up to analyze the direction of the fluid, its displacement that were fundamental vector magnitudes for the analysis of this. Using this prototype, through experimentation, the continuity equation is demonstrated, establishing that no matter the area of a tube, the amount of fluid that passes through will be the same.

Figure 2.

3D Element

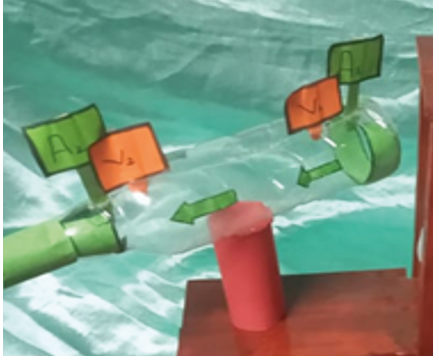
Own Creation

Figure 3D refers to the graphical representation, serving as a tool that facilitates the demonstration of the continuity equation, in which the mass between both tubes is constant, that is, that the mass differential in one tube is equal to the other, being equal to the density times the volume differential. Since the density is constant, only the differential of the volume is taken up, which is equal to the area by the differential of the distance, it is worth mentioning that the distance is velocity times time, this being the same for both fluids (tubes) which travel to the same direction, with the result that by the is equal to the by the. In this way, using the 3D figure, the continuity equation is physically and mathematically demonstrated. área 1 velocidad 1 área 2 velocidad 2

Figure 3.

3D Cube

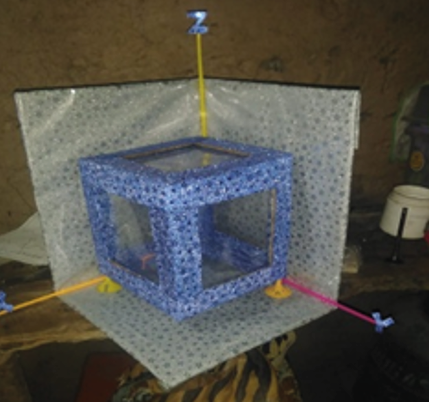
Own Creation

The three-dimensional cube is a graphical representation that illustrates the fluidity of a flow in a closed body in space, considering the three dimensions that emphasize the directions that the fluid should take within a control volume. This representation is an effective resource to mathematically demonstrate the continuity equation in its differential and integral forms, considering the use of vectors. (x, y, z)

The proof of the continuity equation in its differential and integral forms is based on the Reynolds transport principle, which states that in a closed system, no mass enters or exits, implying that there is no loss of mass. The continuity equation is fundamental, as it seeks to conserve the fluid in both space and time, considering a temporal derivative and a spatial derivative.

The choice of a three-dimensional cube as a geometry is because it is the easiest way to comprehend. Importantly, by constructing the three-dimensional cube and performing the differential analysis, detailed information about the behavior of the fluid at each point can be obtained. Comprehensive analysis, on the other hand, encompasses a broader and more general system, but both forms of analysis are equivalent and complement each other.

It is worth mentioning that thanks to this prototype, the importance of linking so many contents of Physics and Applied Mathematics is verified.

Now, when working on the Educational Evaluation part, an evaluation rubric was designed that allows the evaluation of the work carried out on the prototype of experimental practical work for the demonstration of the continuity equation in a differential and integral way, when considering vectors. In addition, co-evaluation, and self-evaluation as a group,

Through the design of the rubric, it was possible to self-evaluate our work, since when it is applied, it will help to improve future research. The rubric contains criteria that will evaluate the general work, both written and experimental. In addition, the criteria proposed are to assess our effort, commitment, and dedication throughout the research process. It also contains punctuation according to the quality of the work done, considering, spelling, writing, and structure.

Therefore, hetero-evaluation helped to identify the difficulties the shortcomings of the work, to adapt the prototype according to the objectives, to avoid repetitions within the same paragraph and throughout the document.

The co-evaluation allowed us to improve responsibility and collaboration at work, identify the achievements achieved both individually and as a group, improve participation, and develop attitudes within the work team.

Based on the performance of the work, it can be said that, as researchers, the results obtained through the process of elaboration of the evaluation rubric was the improvement according to the contents that it has an impact, being of great help for us as trainers and future teachers, since by designing this tool, it will help the development and improvement as future professionals.

Figure 4.

Elements of the Assessment Rubric

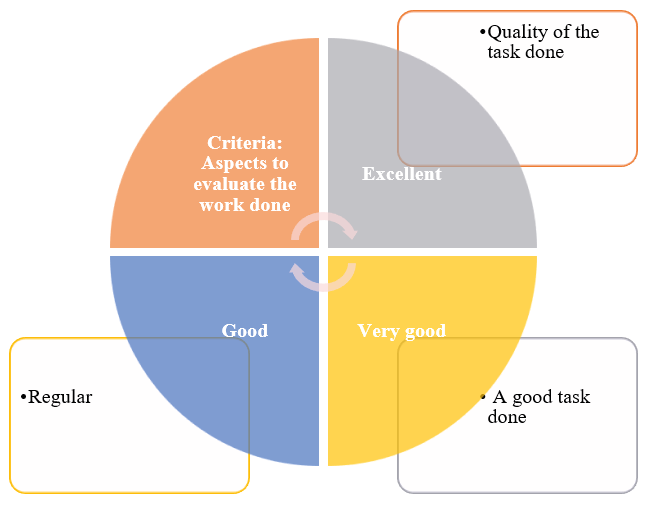
Own Creation, obtained from SmartArt 2022

4. CONCLUSIONS

As a conclusion of this research work, it can be said that it is based on the objectives set; it was shown that, through the prototype of experimental practical work, having been thoroughly analyzed, it was possible to deduce that the continuity equation in its differential and integral form is evident in this prototype.

Therefore, through the experimental practice carried out, it is evident that the principle of continuity is fulfilled, just as the vectors were evidenced to establish the direction of the fluid, that is, the vector magnitudes such as time and velocity. As in the 3D figure, the graphical representation was obtained for the analysis of the continuity equation, such as its elements. In addition, in the 3D cube, the continuity equation was demonstrated in its integral differential form, because it refers to the fluidity of a fluid in a closed body, taking it up, as well as a control volume.

In emphasis, it was possible to demonstrate, the topic of study, through the, that it gives an outlet to each of the objectives, therefore, we proceeded to design the evaluation rubric with which the work done is evaluated, thus allowing to take up aspects to improve in future research. Therefore, in the process of reviewing the brief, it was determined that the approach adopted is qualitative, descriptive, and interpretative. According to the analysis of the information obtained, it is highlighted that the interpretation of the content was carried out through practical experimental work since the continuity equation is met.

It is worth noting the interdisciplinary nature of the research, as different contents are linked to different subjects. Thanks to the performance of this research, it was possible to determine aspects of great importance and to know more about the content of the study.